When you make a trade, what percentage of your capital are you allocating to that trade? This is arguably the single most important variable of the trade, yet it’s commonly ignored by most traders (hence most traders are not profitable).
Take the best trading strategy in the world and apply poor risk management, and you’ll still manage to go broke eventually, so let’s talk about risk management. Specifically, I want to discuss appropriate position sizing for short-term trading.
I see a lot of investors/traders in the crypto space, especially new ones, flounder about multiple strategies such as fundamental value investing, long-term investing, swing-trading, low-cap investing, flipping NFTs, etc. It’s not uncommon to see people FOMO in at the top intending to make a short-term trade, watching as it moves against them, and subsequently say “I’ll just HODL it as a long-term investment.”
Let’s assume for now we’re operating within the confines of a short-term swing trading strategy where positions are usually held anywhere from a few days to a few weeks, so we can discuss position sizing within the scope of said strategy.
Position Sizing
A common rule of thumb is to only risk anywhere between 1% to 3% of your current account balance on any given trade.
Let’s say you average $2,000 (2%)risk per trade in an initial $100k account. This means you’d have to lose 50 trades in a row before blowing the account.
If you were to adjust your risk parameters to 2% of your current account balance, you’d be able to lose a considerably higher number of trades before effectively blowing the account. For example, if you lost your first trade where you risked $2,000, your new current balance would be $98,000 meaning your next trade would risk 2% * $98,000 = $1960, decreasing the rate of drawdown over time.
Kelly Criterion Simplified
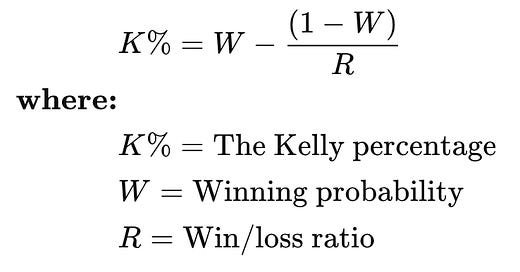
But have you ever stopped to wonder why the recommended risk per trade is roughly 2%? It comes from a math equation known as the Kelly Criterion which allows us to find the optimal risk sizing given your win rate and win percentage.
Before we jump into the math, let’s break this down conceptually with a simpler example. Imagine you have a magic coin that lands on heads 60% of the time and lands on tails 40% of the time. Obviously you should bet on heads every time… But how much should you bet? Let’s say we start with $100. If we bet all $100 on the first coin flip, we have a 40% chance of losing everything.
This is why most new traders will manage to blow their account in a short period of time. Even if they have a good strategy, they bet too much, and a string of a few bad trades destroys their account.
Now what if we bet $0.01 per flip? Well since our expected gain per coin flip would only be 2 tenths of a cent, it would take us 50,000 coin flips to turn our initial $100 into $200! Here’s the math that explains this expected value calculation:
(probability of winning x bet size) - (probability of losing x bet size) = Expected Value
(0.6 x $0.01) - (0.4 x $0.01) = $0.002
$100/$0.002 = 50,000 coin flips.
In other words, on average, we expect to win $0.006 per flip and lose $0.004 per flip for an average gain of $0.002 per flip.
This other extreme (small bet sizing) feeds into the psychology of why traders commonly oversize their positions, and it all comes down to impatience. Many traders want to make money fast and feel that if they do not bet large enough, it will take a long time to see their portfolio grow.
So what is the optimal bet sizing for the coin flip example? We can use the Kelly Criterion formula to find it:
Winning probability = 0.6
Win/loss ratio = 2 (this means our odds are 2:1, or we risk $1 to get make $2 each bet)
K% = 0.6 - ((1 - 0.6)/2))
K% = .6 - (0.4/2)
K% = 0.6 - 0.2
K% = 0.40
So in this coin flip example, we have good enough odds that we should be willing to bet 40% of our current account each time. There’s a 6.4% chance (0.4 x 0.4 x 0.4) of losing 3 times in a row, which would lead to our account drawdown looking like this:
$100 - $40 = $60
$60 - $24 = $36
$36 - $14.40 = $21.60
With our 60/40 mathematical advantage, however, we’d expect that over time we’d be maximizing our expected winnings while minimizing the chances of going broke.
Psychologically, many traders would get impatient after two losing flips here despite having a mathematical advantage. Instead of their third bet being $14.40, (40% of $36), they’d risk blowing the entire account with a final $36 all or nothing bet.
Kelly Criterion Applied
Now let’s apply this formula to a realistic trading strategy where we win 30% of our trades and lose 70% of our trades. Wait!? Wouldn’t that be… unprofitable? Sure, if our R (win/loss ratio aka risk:reward ratio) is 2:1.
But if when we’re right on a trade we win 3x more than we lose when we’re wrong, we have a risk:reward ratio of 3:1, which is now profitable given the above 30% win rate.
Still confused? Let’s take a 10 trade example. We lose 7 and win 3. But when we win, we win $3, and when we lose, we only lose $1 because we use disciplined stop losses:
Win: $3 x 3 = $9
Lose: $1 x 7 = -$7
Profit: $2
This is why our trade strategy should seek to execute trades that strike a profitable balance between risk:reward ratio and win rate.
Now let’s plug this example into the Kelly Criterion formula:
Winning probability = 0.3
Win/loss ratio = 3 (this means our odds are 3:1, or we risk $1 to get make $3 each trade)
K% = 0.3 - ((1 - 0.3)/3))
K% = .3 - (0.7/3)
K% = 0.3 - 0.2333
K% = 0.06667
With this strategy, we should be risking 6.7% of our account per trade. This is actually quite a bit higher than the 2% rule of thumb we discussed earlier, but we really need to consider correcting for any potential bias. First, we often think we’re better traders than we actually are, and until we have a decent sample size of 100+ trades using the same strategy to calculate our real win rate and risk:reward ratio, we should probably air on the side of caution.
The other reason to correct our position sizing down to 2% is the psychological effects of a bad drawdown like we discussed in the coin flip scenario where we lost 3 flips in a row. Are you disciplined enough to continue only risking 6.7% of your account per trade if you had a series of losing trades that drew your account down 40%?
The Kelly Criterion isn’t perfect considering it doesn’t take into account our level of confidence for a given trade, however, it does give a really solid reference point.
If you’re extremely confident in a trade, consider sizing up to your K%, (5 - 7% in this example). If you are moderately confident in a trade, consider taking a sizing of K% divided by 2 or 3, so 2% - 3% in this case. And if you are less confident, consider sizing down even further, or not taking the trade at all (why take a trade you aren’t confident in).
That’s it for this newsletter, hope it helped!
Remember to follow me on Twitter: @OxStacker
…and check out CryptoStackers Pro if you haven’t already!